Page 406 - môc lôc

EXPLANATION OF TERMINOLOGY, CONTENT AND
METHODOLOGY OF SOME STATISTICAL INDICATORS
ON PRICE

Consumer price mentions the expense of consumers for a unit of
commodity or service to serve their daily lives. Consumer price shows the
retail price of goods on the market or the cost of services for people’
livings. In case, commodities are not priced and can be bargained,
consumer price is the final price of commodity paid by consumers.

Consumer price index (CPI) is a relative indicator (%) reflecting
the tendency and change over time in the prices of consumer goods and
services purchased by people.

The representative list of goods and services for measuring CPI
consists of key goods and services which represent for the population’s
consumption in a certain period.

Weight for CPI compilation is the expenditure share for goods and
services groups in the total of population’s expenditure in base year.

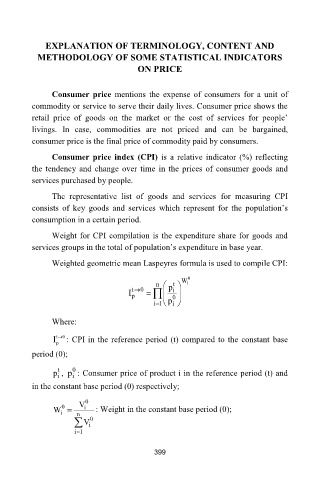
Weighted geometric mean Laspeyres formula is used to compile CPI:

n  p t  W i 0

I t 0    i 0 

p
i 1 p i 
Where:

I t 0 : CPI in the reference period (t) compared to the constant base
p
period (0);

t
0
p , p : Consumer price of product i in the reference period (t) and
i
i
in the constant base period (0) respectively;
W i 0  n V i 0 : Weight in the constant base period (0);
V 0
 i
i 1

399